Fractal Images

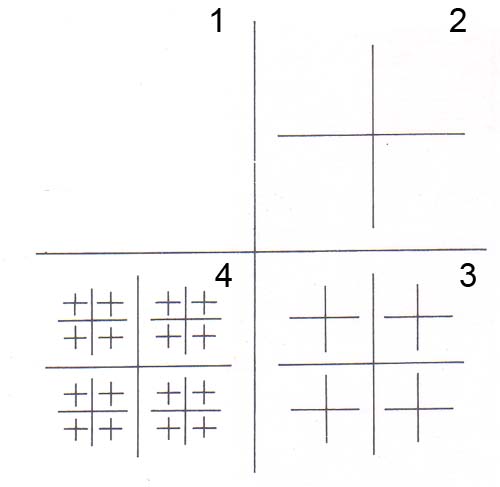
Factals are geometric figures that are self-similar at all scales. The branchings of trees and lightning are fractal forms. Benoit Mandelbrot, a mathematician working at IBM, discovered a way of producing fractal images by means of iterating equations and plotting the results on a computer terminal. During the 1990s many computer buffs wrote software to make fractal images. Such images can be strikingly beautiful, if a bit bizarre. They are often somewhat askew in shape, although they can also be perfectly symmetrical. The intricacy of many images recalls the complicated designs of Celtic knot patterns, the illuminations of the Lindisfarne Gospel and the Book of Kells, Islamic tilings, and other ornamental designs that exhibit what art historian/psychologist E.H. Gombrich has termed amor infiniti--a love of the infinite. In The Sense of Order Gombrich provides an illustration of what he calls decorative filling. The crosses in this illustration can be repeated at smaller and smaller scales. Utimately, the repetitions will fill the originally blank space. Crosses within crosses within crosses....

Nearly all books or web pages about fractals have an image of the Sierpinski Triangle, which fills its space in a manner similar to Gombrich's crosses.

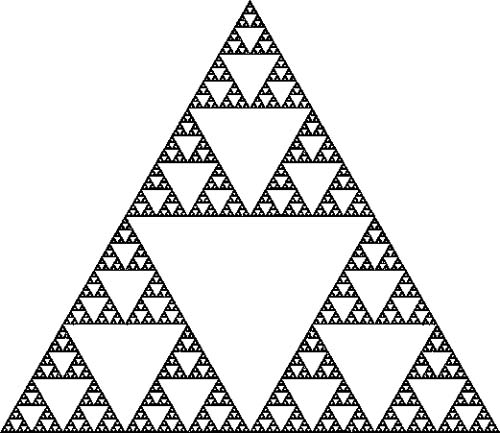
Triangles within triangles within triangles....

Below is a sampling of some of the images I produced with software that I wrote.

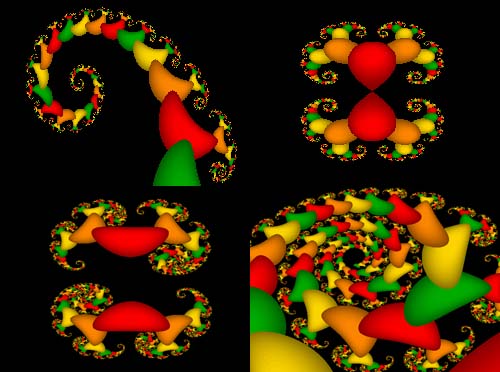
Here spirals extend from spirals as far as pixel resolution allows. By this I mean that if we blow up a small section of any of these four images, a pattern of sprials on spirals appears. The blow-up resembles the larger pattern we began with. Mathematically a fractal is infinitely extensible. Although we can see only a part of the infinity of self-similar patterns, this glimpse is sublime.

I call this one "Firedance." The spiky shapes come from a method of fractal image production called orbit trapping. Orbit traps greatly enhance the weird visual aspects of fractals. "Firedance" is derived from a Julia fractal. Here is what an unenhanced Julia fractal looks like.

This image is quite striking, but it does not have the globular, or ovate, or tubular, or spiky appendages that the orbit trapped images above possess.